Boardgame Maths – Kingdomino

By David Banham (June 2019)

I am not sure whether my love of maths was inspired by my love of games and puzzles or vice-versa. What I do know, is that I am always fascinated by the intersection of these two great things in the Venn diagram of my life.

A recent example is the game Kingdomino by Blue Orange games. The game, which won the Spiel des Jahres in 2017, is beautiful in its elegance and simplicity but has an intriguing mathematical coincidence at the heart of its design.

Before we get into the deeper mathematical coincidence, it is worth stopping to appreciate the actual mathematics involved in the gameplay, which in itself, provides a fantastic platform for practising basic numeracy skills in a fun and rewarding way.

At the start of the game each player selects a 1 x 1 castle tile that acts as the centre of their kingdom. Players then take turns to select a domino of dimension 2 x 1 from a shared pool, adding each domino to their kingdom, with the aim of making a 5 x 5 square from the pieces selected. There are 49 domino tiles in the game and, rather than having numbers, each depicts up to two kinds of terrain type (either mountain, pasture, sea, swamp, field or forest). In addition, some of the dominos depict buildings that are worth either 1, 2 or 3 crowns. Unlike conventional dominos, it is not necessary to match terrain type to terrain type when adding dominos to your kingdom, however, you score points at the end of the game by multiplying the number of crowns in each connected region of the same terrain type by the number of squares in that region.

For example, in this partially completed game I am currently scoring 21 points. This breaks down as follows:

3 x 6 = 18 for the pasture land

(3 crowns x 6 matching connected terrain types).

1 x 3 = 3 for the sea

(1 crown x 3 matching connected terrain types).

0 x 3 = 0 for the forest

(0 crowns x 3 matching connected terrain types).

The starting crown tile (yellow/grey) never contributes towards scoring.

You can see straight away how the game has some mathematical merit for practising basic arithmetic, particularly when deciding the best domino to select next.

In the case below, is it better to add to my kingdom by selecting the pasture and forest domino and placing it at the top left, or the sea and forest domino and placing it at the top right?

Well the pasture/forest domino adds an extra 3 points, but the sea/forest domino adds an extra 5 points, despite occupying a smaller area and having fewer crowns in the region. Of course, strategically there are other concerns: should I gamble on enlarging a region (or conversely closing off a region) with no or few crowns in the hope of picking up a double crown domino later in the game? Should I add crowns anyway and hope to expand later? What if I cannot complete my 5 x 5 grid? – poor placement might mean that I cannot place my last domino as it simply won’t fit within the required 5 x 5 footprint!

With this elegant and simple maths, you can see how I have been able to use the game to practise basic arithmetic skills with pupils at both KS2 and KS3, but, what really intrigued me about the game design was the choice of 48 dominos.

It seemed obvious at first:  with 48 dominos you can cover 96 squares (48 x 2 as each domino is made of two 1 x 1 squares). If you then include a start tile (each of size 1 x 1) for each of the 4 players in the game you have a total area of 100 squares in the game, enough for each player to make a 5 x 5 kingdom (4 x 25 = 100). However, what I found really fascinating, was that the game can still be played with a full set of dominos as a 2 player game if each player makes a 7 x 7 kingdom instead.

Not convinced? Let’s do the maths:

Using all the dominos you still cover 96 squares (48 x 2), but in a two player game you only use 2 start tiles, making a total coverage of 98 squares in the game. If each player makes a 7 x 7 grid that’s 49 x 2 = 98 squares.

It is this interesting link between square numbers that really got my mind thinking. Did the designer plan this? Or was it a happy coincidence? What other domino counts would enable us to play the game as both a 2 player and 4 player game, still making square kingdoms?

Time for some real maths …!

For the game to work at both player counts we would need the number of squares available to every player in both a four player game and a two player game to be a square number.

Let n = the number of dominos in the game

Then in a 4-player game the total number of squares in play would be 2n + 4

(Each domino covers 2 squares, plus there are 4 start tiles).

This means that each player would have to build a kingdom that covers    squares (as the total number of squares in play would need to be shared between all 4 players).

We need this value to be a square number.

In other words we need  for some positive integer ‘p’, and each player’s kingdom would be of size p x p.

In a 2-player game the total number of squares in play would be 2n + 2

(Each domino covers 2 squares, plus there are 2 start tiles).

This means that each player would have to build a kingdom that covers  squares (same as before but shared between 2 players only).

This number also needs to be a square number.

In other words we need      for some positive integer ‘q’, and each player’s kingdom would be of size q x q.

We have these two equations that must be satisfied at the same time:

           Eq1

          Eq2

Rearranging each equation gives:

2n + 4 = 4p²                                        2n + 2 = 2q²

Combining both equations to eliminate 2n:

2 = 4p² – 2q²     

1 = 2p² – q²

This tells us that for the game to work we would need the size of the four player kingdom (p²) to be 1 more than the size of the two player kingdom (q²) when doubled.

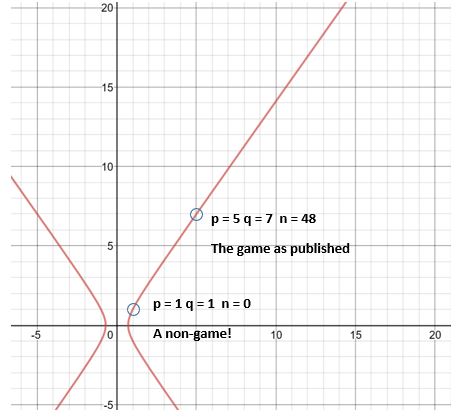
My number theory is not good enough to deduce how likely it is that two such square numbers can be found, but we can see straight away that a 5 x 5 four player kingdom (p = 5) and a 7 x 7 two player kingdom (q = 7) will work since:

1 = 2 x 5² – 7²

Substituting either of these values into Eq 1 or Eq 2 and solving for ‘n’ gives a value of 48 (the number of dominos in the game).

By just substituting in values it is pretty quick to spot that p = 1 and q = 1 also works, however this would lead to a very dull game as players at both player counts would require n = 0 (ie no dominos!) – they would merely place the start tile and everyone would score zero points!

A bit more trial and error got me no-where, so I resorted to Desmos, graphing the equation 1 = 2p² – q² and searching for integer values on the curve by eye!

This gave a very pleasing graph:

Which nicely confirmed the two cases I had already found, but didn’t make searching for another much easier.

Eventually however I found one! p = 29 and q = 41

Substituting these into Eq1 and Eq2 confirmed that n = 1680 meaning that if you had 1680 dominos you could play a 4 player game making squares of 29 x 29 and a 2 player game making squares of 41 x 41 (this is a lot of cardboard!)

I didn’t find any other solutions – although I am confident that there will be more. The closest I got was p = 99 and q = 140 which almost require the same number of dominos (19600 for the 4 player game and 19599 for the 2 player game), but I guess if you were playing with that many dominos the scoring at the end would definitely be a chore rather than an exercise in numeracy!

Note: Kingdomino is a 2 – 4 player game and you can play the game at all player counts using a 5 x 5 grid, by reducing the number of dominos available at the start of the game.

Note: Although I think the game is great I have no connection with Kingdomino or Blue Orange games!

.